Electron on the scale
A measurement of electron mass which is more precise by a factor of 13 could have an impact on the fundamental laws of physics
Electrons are the quantum glue of our world. Without electrons there would be no chemistry, and light would be unable to interact with matter. If electrons were only a little heavier or lighter than they are, the world would look radically different. But how can a particle which is so tiny that it has so far been considered point-like actually be weighed? This feat has now been achieved in a collaborative project involving physicists from the Max Planck Institute for Nuclear Physics in Heidelberg who "weighed" the mass of the electron 13 times more precisely than previously known. As electron mass is involved in fundamental physical constants, it is of significance to fundamental physics.

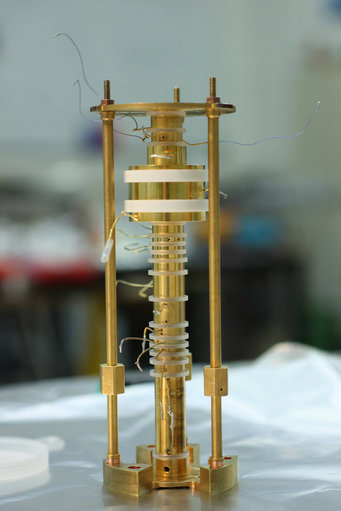
"Normally, you would need to carry out ten or twenty years of research in precision physics to improve a fundamental value by a single order of magnitude", says Klaus Blaum, Director at the Max Planck Institute for Nuclear Physics in Heidelberg, who is overjoyed to report the "huge response" that this most recent result is generating at scientific conferences. In just a few years, the collaborative research, headed by the Heidelberg team, has managed to determine the value for the mass of an electron more precisely by a factor of 13. Project leader Sven Sturm explains the extremely high sensitivity of the "scale" used to achieve this result with the following image: "If we were to apply this to an Airbus A-380, we would be able to detect a mosquito as a stowaway just by weighing."
The fact that physicists now know the mass of the electron to eleven decimal places is important, because electrons are pretty much ubiquitous. Just reading this text means that electrons must convert light into nerve impulses in the eyes. These minuscule particles, which, according to current knowledge, have no spatial extent, thus have immense power in nature. Their mass is linked to, among other things, the value of fundamental physical constants. One example is the "fine-structure constant" which determines the shape and properties of atoms and molecules. "It basically describes everything we can see", says Blaum, "because it plays a central role in the interaction between light and matter." If nature had given electrons an only slightly different mass, atoms would look completely different. Such a world would probably be very strange.
Electron mass is measured together with a carbon nucleus
The mass of the electron is also a central variable in the so-called standard model of physics, which describes three of the four currently known fundamental forces of physics. Although the model works impressively well, it is now nevertheless clear that there are limits to its validity. Where the limits of the standard model lie however, is an open question. Precise knowledge of the mass of the electron can thus be of vital assistance in the search for previously unknown physical interrelationships.
The team of physicists headed by Klaus Blaum and Sven Sturm developed an ingenious experiment in order to determine the electron's extremely small mass. Weighing, in principle, requires a reference for comparison. "If you get on the scales in the morning, in old mechanical models it is a spring", explains Blaum. Beam scales have a counterweight as reference. In the case of the electron, the physicists faced the problem that all fundamental particles, which could meaningfully be used as reference weights, are much heavier. "The proton or neutron, for example, is two thousand times heavier", explains Blaum, "which would be like trying to weigh a rabbit with an elephant as the counterweight." So for their experiment, the physicists made use of a cunning strategy. Although they brought two vastly unequal masses together, they didn’t even try to weigh the electron rabbit directly with the help of an atomic elephant.
Sven Sturm, Blaum's doctoral student at the University of Mainz, set up the experiment. "The main challenge was to develop the measurement method", he says. As a post-doc, he went on to head the team which carried out the precise measurement of the electron mass. The physicists paired a single electron with a bare nucleus of the immensely heavier carbon (C) 12 isotope. This carbon isotope was chosen with careful consideration, as it is the basis for the atomic mass unit. The mass of C 12 is by definition exactly known and using it as a reference excludes a major source of error. "Controlling systematic error is absolutely vital", emphasises Sturm.
Penning trap sets the carbon ion racing around a circuit
The physicists blasted off five of the carbon atom's six electrons in order to prepare a C12 nucleus with a single electron. The remaining carbon ion with a charge of five - the carbon nucleus with a single electron - was set racing around a circuit which, viewed in a highly simplified way, can be thought of as circular. A so-called Penning trap with its extremely uniform magnetic field forces the carbon ion to follow this circular path.

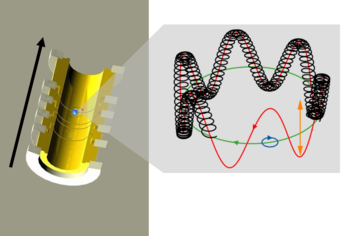
How can an electron be weighed? In a Penning trap (left), a magnetic field (black arrow) forces a carbon 12 nucleus with a single electron to follow a helical course (right). Viewed in a simplified way, this circuit can be thought of as a circular path (green). The precise mass of the carbon 12 nucleus with one electron can be determined on the basis of the revolution frequency. The mass of the electron is then obtained by using quantum mechanics to relate the mass of carbon atom with its charge of five to the precession of electron spin (black line, right).
"The aim, when making precision measurements, is always to make the measured variable precisely countable", says Blaum, as he explains the thinking behind the method: "In a Formula 1 race on a circuit, spectators can count how many times a racing car shoots past and, if they know the length of the circuit, can estimate the car's speed." The situation is similar in a Penning trap; however in this case, the physicists were able to measure even the tiniest fractions of complete circuits.
Quantum mechanics was of assistance in the second step, which was then required for determining the mass of the electron. Electrons have what is known as "spin" that makes them act like tiny magnets. In the strong magnetic field of a Penning trap, this spin precesses or wobbles like a tiny gyroscope. Although this precession is extremely rapid, the physicists had a strategy to measure it precisely. The key is that the revolution frequency of the carbon ion in the trap and the wobble frequency of electron precession are in an exact ratio to one another. Like a gear mechanism, quantum mechanics links the mass of the carbon ion firmly to the mass of the electron, which is thereby measurable.
Only a theoretical contribution enabled the measurement of the electron mass
There was, however, a rather poorly understood "gearwheel" in the mechanism known as the g factor or gyromagnetic factor. "This was where our close cooperation with Christoph Keitel's Theory Group at our Institute played a vital role", explains Blaum. On the basis of previous results from the same collaboration, the Institute's theoreticians, led by Group Leader Zoltán Harman, were able to calculate the g factor more precisely than in the past, thus enabling an exact determination of the electron mass.
Such high-precision experiments benefit from a collaborative approach with scientists who can contribute different expertise. Physicists from the GSI Helmholtzzentrum für Schwerionenforschung in Darmstadt and the Johannes Gutenberg University Mainz made significant contributions. The result is a fantastically precise number revealing that an electron has a mass 1/1836.15267377 that of a proton. Stated in kilograms, the electron's mass is around an unimaginable 10-30 kilogram, or thirty zeros after the decimal point. Although the electron truly is a lightweight, it plays a heavyweight role in nature.
RW/PH